4.6 Inverse Trigonometric Functions
Principle trigonometric functions
Since the trigonometric functions are not one-to-one functions, they do not have inverse functions in the customary sense.
In order to define inverses of the trigonometric functions, it is necessary to restrict their domains to a subset on which they are one-to-one.
An inspection of the graphs of the six trigonometric functions reveals that they are one-to-one on the following domains:
- Sine \([ -\frac{\pi}{2},\frac{\pi}{2} ]\)
- Cosine \([ 0, \pi ]\)
- Tangent \(( -\frac{\pi}{2},\frac{\pi}{2} )\)
- Cosecant \( [ -\frac{\pi}{2} , 0 ) \cup ( 0, \frac{\pi}{2} ] \)
- Secant \( [0, \frac{\pi}{2} ) \cup (\frac{\pi}{2}, \pi ] \)
- Cotangent \((0, \pi )\)
The inverse trigonometric functions are found by inverting just the portion of the corresponding trigonometric function on the intervals specified above. Angles lying in the specified intervals are called the principle angles for the function.
Exercise 4.6.1
Sketch each of the six trigonometric functions on the intervals specified above.
See SolutionNotation and caveat
The inverse trigonometric functions are denoted by \(\sin^{-1} x, \cos^{-1} x, \tan^{-1} x, \csc^{-1} x, \sec^{-1} x, \cot^{-1} x\) or as \(\arcsin x, \arccos x, \arctan x, \text{arccsc } x, \text{arcsec }x, \text{arccot }x\).
For customary inverse functions, both \(f ( f^{-1} ( x ) ) = x\) and \(f^{-1} ( f ( x ) ) = x\) are true, but for inverse trigonometric functions, it is true that \(f ( f^{-1} ( x ) ) = x\) , but it is not generally true that \(f^{-1} ( f ( x ) ) = x\) unless \(x\) is a principle angle for that function.
For example, \(\sin^{-1} ( \sin ( 2\pi ) ) = 0\), not \(2\pi\) since \(2\pi\) is not a principle angle for the sine function. The principle angles for sine consist of all angles in the interval \([ -\frac{\pi}{2},\frac{\pi}{2} ]\) and \(2\pi\) does not lie in that interval.
The symbols \(\sin^{-1} ( \sin ( 2\pi ) )\) translated into words say "The principle angle whose sine is the same as the sine of \(2\pi\)". And that principal angle is \(0\), not \(2\pi\).
Here are two more examples:
- Find \(\arctan\left(\tan\left(\frac{3\pi}{4}\right)\right)\). Solution: The principal angle (for tangent) having the same tangent as \(\frac{3\pi}{4}\) is \(-\frac{\pi}{4}\).
- Find \(\sec^{-1}\left(\sec\left(\frac{3\pi}{4}\right)\right)\). Solution: Since \(\frac{3\pi}{4}\) is a principle angle for secant, the answer is \(\frac{3\pi}{4}\).
Trigonometric functions of inverse trigonometric functions
In Exercises 4.5.6 and 4.5.7 you used the Right Triangle Method to find all the trigonometric functions of an angle \(A\) given the value of one of the trigonometric functions of \(A\) and the quadrant containing the terminal side of \(A\). The next exercises are the same type of problem in different clothing. The key to the next exercises is the fact that the outputs of all the inverse trigonometric functions are principal angles. Thus, the expression \(\tan^{-1}( - 3 )\) means the principal angle whose tangent is \(-3\).
Example: Find \(\sin ( \tan^{-1} ( - 3 ) )\). That is, find the sine of the principle angle whose tangent is \(-3\). Now the principle angles for tangent must lie between plus and minus \(\frac{\pi}{2}\). If the tangent is negative, then the principle angle must be in quadrant IV. Call the principle angle \(A\) and label the triangle as follows:
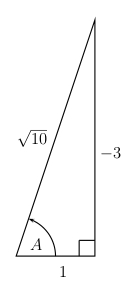
Thus, we can see that \(\sin ( \tan^{-1} ( - 3 ) ) = -\frac{3}{\sqrt{10}}=-\frac{3\sqrt{10}}{10}\).
There is a useful rule of thumb that can be used when placing negative signs on the triangle. This rule of thumb is valid only when working with principal angles.
The negative sign always goes with the numerator unless that would place it on the hypotenuse
.Notice in this last example, we placed the negative with the \(3\) instead of with the \(1\). That is because the tangent of \(A\) is the opposite side divided by the adjacent side. The opposite side is the numerator for the tangent function. So the negative goes on the opposite side.